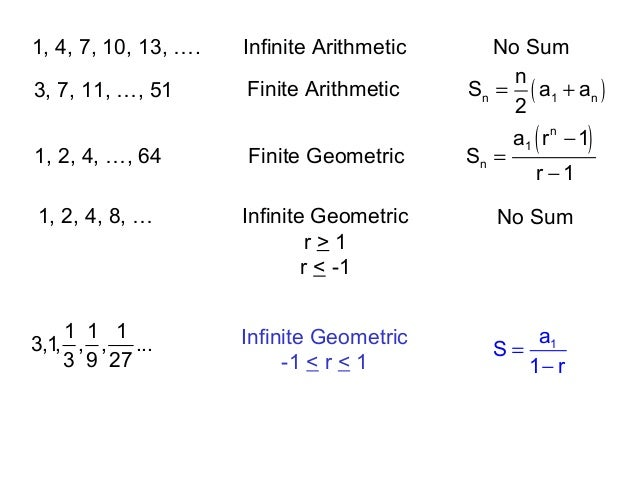
Our method for shifting the exponent in Steps (3) and (4) may cause some confusion for students. In the above solution, we started by changing the index of summation (students may want to, as an additional exercise, try to solve this problem by first changing the exponent). To convert our series into this form, we can start by changing either the exponent or the index of summation. Students should immediately recognize that the given infinite series is geometric with common ratio 2/3, and that it is not in the form to apply our summation formula, Possible Mistakes and Challenges Getting started Absolute Convergence Implies Convergence.The Contrapositive and the Divergence Test.A Motivating Problem for the Alternating Series Test.Example: Integral Test with a Logarithm.A Second Motivating Problem for The Integral Test.A Motivating Problem for The Integral Test.Final Notes on Harmonic and Telescoping Series.Videos on Telescoping and Harmonic Series.Introduction: Telescoping and Harmonic Series.Example: Properties of Convergent Series.Videos on the Introduction to Infinite Series.A Geometric Series Problem with Shifting Indicies.Converting an Infinite Decimal Expansion to a Rational Number.Example Relating Sequences of Absolute Values.Relationship to Sequences of Absolute Values.
Convergence of Infinite Sequences Example.